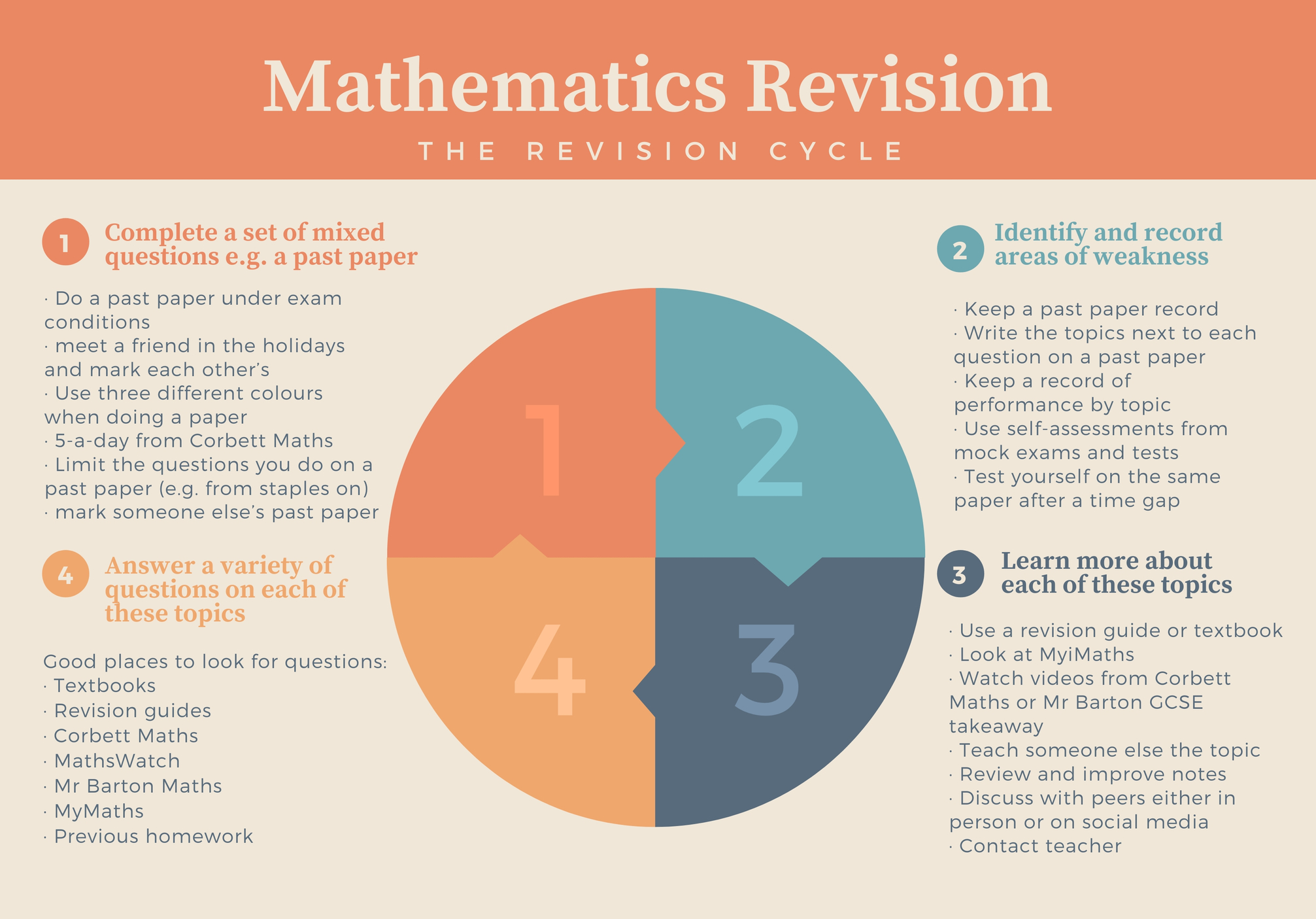
The Revision Cycle
Written by Jocelyn. Jocelyn is available for private tutoring!
Most students don’t actually know how to revise. They re-read their notes and recognize the content, and practice recognizing until they become really rather good at it. Unfortunately, almost no tests ask if you recognize the nth term of a linear sequence. They ask you to find the nth term of a linear sequence, and this is a very different skill indeed.
The work of UCLA based cognitive scientists Robert and Elizabeth Bjork and the “What makes great teaching” report highlight (you’ll get the joke if you’ve read it) the same problems with revision, and give some suggestions about the most efficacious revision with a real focus on the need for “Retrieval Practice” (Landauer, 1978). Unlike recognizing, Retrieval Practice requires you to answer a question and therefore retrieve the information or skill. Exams are based on retrieval; revision should be as well. A few years ago, I created “The Revision Cycle”, which combines evidence-based techniques for memorizing with motivational theory and my experience of what works well for time-poor students who want to succeed in a wide range of endeavors.
The basic premise is that most students don’t use past paper practice efficiently. They often bounce from past paper to mark scheme and get the same type of questions wrong on each paper, because no real learning takes place between each attempt. In a perverse way, scoring 70% on a past paper means that you just wasted 70% of the time spent doing the paper, because those were things you already knew how to do. How much better off would you be if all of that time had been spent learning material that you didn’t know?
Learning things you don’t already know is hard. It is much easier to do a past paper, get most of it right, and convince yourself that you could have done the questions you got wrong by agreeing with the answers on the mark scheme, but it rarely leads to real improvement and is highly inefficient. The Dunning-Kruger effect explains how novices incur a “double burden-not only does their incomplete and misguided knowledge lead them to make mistakes but those exact same deficits also prevent them from recognizing [sic] when they are making mistakes” (Dunning, 2011).
This makes reading through a mark scheme or a set of answers quite a dangerous exercise as it leaves you believing you know a great deal more than you do. When learning a foreign language, comprehension is always at a higher level than speaking, because while comprehension is an exercise in recognition, speaking requires retrieval. Indeed, this is why I try to avoid issuing my students with “topic lists” before tests. While it makes a great deal of sense to triage your revision so that you spend the most time on the topics that need it most, doing so from a topic list is unlikely to be effective because rather than determining whether you can retrieve the required knowledge, you are making your competence assessments based on whether or not you recognize the topic.
So what is The Revision Cycle? Here is one I made for my GCSE Mathematics group, but the structure would work well for many other subjects and qualifications.
The Revision Cycle starts in quadrant 1, by completing a set of mixed questions. Next, the student uses the results from the mixed questions to identify the topics where knowledge is least secure. Quadrants 3 and 4 are where the real learning happens, and ensure that time is used most efficiently.
As an example, consider Charlie, who is revising for his IB® Higher Level Mathematics exam. Charlie starts his revision by completing a past paper (Quadrant 1). He marks the paper and identifies that he got questions wrong on proof by induction, complex numbers, integration by parts, and the Chi-Squared test (Quadrant 2). When Charlie is going through the answers, he understands what he should have done, but Charlie knows this is recognizing not retrieving, so he is not fooled into thinking he is ready to move onto another past paper. Instead, he makes a list of these topics and addresses each topic. He re-watches a video about proof by induction (Quadrant 3), then answers questions from this chapter in his textbook (Quadrant 4). He asks his older sister to explain complex numbers to him (Quadrant 3), then he re-does a quiz his teacher had given on the topic (Quadrant 4). For each topic identified from this past paper, he re-learns the material and answers more questions on it. Once he has done this for every topic, he goes back to Quadrant 1 and tries another past paper, beginning another revolution through the cycle.
During the first few cycles, students spend most of their time in quadrants 3 and 4 as early on in their revision, a set of mixed questions reveals quite a few topics which need to be learned and practiced. Only once each topic has been studied critically and practiced, does he attempt another set of mixed questions. So the first loop around the cycle takes the longest, but as more topics are identified and mastered, performance on the mixed questions improves, which means that fewer topics are identified, studied, and practiced. This acceleration around the cycle means that as the exam is approaching, the students are spending almost all of their time on past papers; and getting them mostly correct!
I am utterly convinced that this is the most effective way to revise for mathematics, and quite possibly other subjects too. Many of my students have used The Revision Cycle to great success. Below are a few of their accounts.
Will Feasey is studying Physics at the University of Oxford.
For someone who has always found maths relatively easy, when trigonometric equations hit in the spring of year 11, I was at a loss. Thankfully, my then maths teacher had just released her revision cycle and so I set about looking to overcome this stumbling block. Having identified this area of weakness, I looked over a number of different resources, from teacher notes to online videos. Another method of revision I found to be super helpful was working through problems with friends, sharing our different perspectives, and adapting them all into my own game. By the time it came round to trying another past paper, I was ready to overcome any trigonometrical trickery that was thrown at me. However, as one door was fixed up, another burst open, this time in the guise of the application of calculus to kinematics. In fact, I believe due to the very inherent nature of maths, this cycle is going to carry on for as long as I’m in education. Like taking apart a Russian doll, each year I build on the last’s content, going deeper and deeper and gaining a more fundamental appreciation for the intricacies of mathematics.
As A-levels rolled around, our mock exams looked to expose any weaknesses; the resulting flare-up came from statistical distributions, specifically the Gaussian. Once again, I looked to follow The Revision Cycle, improving my notes on the topic by going along to extra revision classes and practicing a variety of questions from both textbooks and past papers. Even today at university I employ The Revision Cycle to keep on top of the difficult topics. During my first set of examinations, surmising the year’s work so far, a particular area of the issue was special relativity, a subject that utilizes a fair bit of mathematics. Once more I returned to the cycle, first educating myself on the topic from a range of sources and then attempting further problems to really test my understanding.
I expect to keep coming back to this revision cycle for the remainder of my degree and perhaps in the future, I’ll be able to pass on this technique to the next generation of maths students.
George Holding is studying Chemistry at the University of Oxford
I studied Physics, Chemistry, Mathematics, and Further Mathematics at A-level, and during my GCSE years at school, I was first introduced to The Revision Cycle.
I always use an approach similar to the one outlined in The Revision Cycle. There is no point in going over topics that you can already comfortably answer exam questions on, so I will start with something to gauge where my understanding is poor, like a past paper or problem sheets. From here, marking very harshly, I’ll go over the questions, picking out where I went wrong. For me, this is more important than actually doing the paper, and trying to be really picky and mark effectively is crucial to get that exam technique spot on.
I always have a sheet of paper for each subject I’m revising, on which I’ll write down bullet points of the specific topics and types of questions I have struggled with, to go over them. When I go over these sheets, I’ll normally leave time between looking over each bullet point so I retain the information better, rather than trying to cram them, and not really understanding each one. I go over each specific topic in different ways, depending on what it is and how much I already understand it, e.g. if I got a knowledge-based question wrong, I’ll make little cards with questions on one side and answers on the other to test myself, but if it’s an understanding-based one, I’ll watch videos, go through my notes again, etc. Finally, I’ll always come back to a topic after a few days to review my understanding by doing some more questions, and I’ll only cross it off my list if I’ve done well on these.
Thomas Varnish is studying Physics at Imperial College London
I first learned about The Revision Cycle when I was taught A-Level Mathematics. Previously, during my GCSEs, I’d focused solely on completing as many past papers as I could, ultimately wasting a good portion of my time covering questions I was already confident in answering. Since then, throughout my A-Levels and Physics degree, I’ve found the easy-to-follow, structured approach of The Revision Cycle to be essential when preparing for exams.
The Revision Cycle has been far more efficient than any other technique I’ve tried, especially if I haven’t been recently studying a topic. By first attempting a mix of questions, I’m able to rapidly identify any weaker areas, then focus my revision. Additionally, it helps by familiarizing myself with the general exam structure and exam-style questions. I found The Revision Cycle particularly rewarding when revising for my A-Level Mathematics statistics module. I’d begun my revision by completing a few past papers, repeatedly achieving similar grades. Since I didn’t appear to be improving, I tried The Revision Cycle, quickly discovering I was consistently struggling with hypothesis testing questions in each paper. Having identified this weakness, I returned to my notes and textbook, focusing on hypothesis testing. Returning to the past papers, this targeted approach not only helped me to improve my grade but also gave me more confidence for sitting the exam. My revision became more streamlined, and I had a series of clear steps to achieve my goals.
Having now adopted The Revision Cycle throughout my degree, I’ve found combining it with spaced repetition (reviewing the topic after a day, then if I’ve answered all the questions correctly, repeating after another two days, etc.) helps me to ensure I’ve properly understood a subject rather than temporarily recognizing the concept from what I’ve read in my notes. Similarly, if I’m struggling with a question because I’ve forgotten an equation, I’ll look it up in my notes, and use spaced-repetition flashcards (such as the AnkiWeb website) to memorize it.
Dunning, D. (2011). The Dunning–Kruger Effect: On Being Ignorant of One’s Own Ignorance. In: J. Olson and M. Zanna, eds., Advances in Experimental Social Psychology. Academic Press, pp.247–296.
Landauer, T.K, & Bjork, R.A (1978). Optimal Rehearsal Patterns and Name Learning. In: M.M. Grunebert, P.E, & R.N. Sykes, eds., Practical Aspects of Memory. Academic Press, pp. 625-632, Academic Press, London
Warp Drive Tutors, Inc. has been developed independently from and is not endorsed by Cambridge Assessment International Education. A-Level, GCSE and iGCSE are registered trademarks owned by Cambridge Assessment International Education.
Warp Drive Tutors, Inc. has been developed independently from and is not endorsed by the International Baccalaureate Organization. International Baccalaureate®, Baccalaureat International®, Bachillerato Internacional® and IB® are registered trademarks owned by the International Baccalaureate Organization.